Solving Rational Equations: Relationship to Graphs
Purplemath
-
Solve the following equation:
To figure out the common denominator for these fractions, I'll first need to factor that quadratic in the denominator on the right-hand side of the rational equation. This will also allow me to find the disallowed values for this equation. Factoring gives me:
x2 − 6x + 8 = (x − 4)(x − 2)
The factors of the quadratic on the right-hand side "just so happen" to be duplicates of the other denominators. This often happens in these exercises. (So often, in fact, that if you get completely different factors, you should probably go back and check your work.)
Content Continues Below
So the lowest common denominator for this equation will be (x − 4)(x − 2), and I'll need to remember (at the end of my work) that x ≠ 2, 4.
Method 1: I can convert everything to the common denominator and then solve the numerators:
(x2 − 4x) + (x − 2) = 2
x2 − 4x + x − 2 = 2
x2 − 3x − 4 = 0
(x − 4)(x + 1) = 0
x = 4 or x = −1
Method 2: On the other hand, I can multiply through on both sides by the common denominator, and then solve the resulting equation. I've used color below to highlight the bits that cancel off:
x(x − 4) + 1(x − 2) = 2
x2 − 4x + x − 2 = 2
x2 − 3x − 2 = 2
x2 − 3x − 4 = 0
(x − 4)(x + 1) = 0
x = 4 or x = −1
By either method, I get the same result: x −1, 4.
However, when I check these solutions against the denominators of the original equation, I see that "x = 4" would cause division by zero, so I throw that solution out. Then my final answer is:
x = −1
Content Continues Below
I've mentioned previously that the solution process for rational equations can create solutions that don't actually exist. This is because the process, at some point, gets rid of (or at least ignores) the denominators. When this happens, we've done what is called "an irreversible step". That is, we've done something that gets rid of information, and there's no way to get it back (other than returning to the beginning again).
We can see the results of this "irreversible" step graphically.
When solving these rational equations like these, we can think of ourselves as trying to find the intersections of the related functions on either side of the "equals" sign. For instance, let's return to the equation we just solved:
Let each side of the equation be its own function:
Graphing these, we can see that the two functions intersect in one spot:
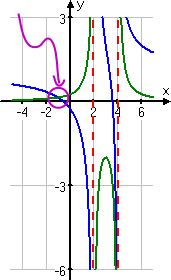
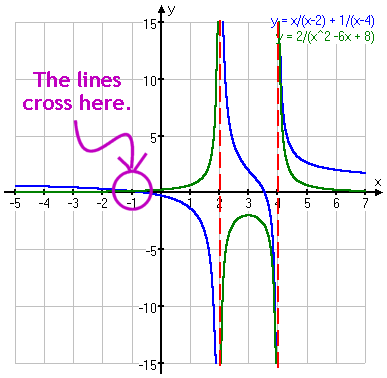
This one spot where the two functions intersect is the solution, x = −1, that we found earlier for the original rational equation.
If you have a graphing calculator, you can check your answers by doing a quick graph, because the solutions to the equations are the intersections of the graphs of the related functions. Do the graphs, and confirm that your solution values match the x-values of the intersection points on the graphs. Or, if they don't match, you know you need to check your work!
But remember how we intially came up with two solutions? That was because we'd gotten to a stage where we were ignoring the denominators. If we ignore the denominators, we get the following new functions:
y3 = x2 − 3x − 2
y4 = 2
These new functions graph like this:


As you can see, the irreversible step of getting rid of the denominators not only eliminated the vertical asymptotes, but it also created an additional (and wrong) solution.
Because this irreversible step is susceptible to the creation of extraneous solutions, you must always check your solutions for rational equations against the original equation's denominators, throwing out any solutions that would cause division by zero.
Affiliate
Affiliate
Solving rational equations is pretty straightforward if you are careful to write each step completely. But (warning!) as soon as you start skipping steps or doing stuff in your head, you're going to start messing up. So always work neatly and completely. And never forget to check your solutions, because I can just about guarantee that you'll have one of those "no solution" (or "only one of these solutions actually works — ha, ha!") problems on your next test.
You can use the Mathway widget below to practice solving a rational equation. Try the entered exercise, or type in your own exercise. Then click the button to compare your answer to Mathway's.
Please accept "preferences" cookies in order to enable this widget.
(Clicking on "Tap to view steps" on the widget's answer screen will take you to the Mathway site for a paid upgrade.)
URL: https://www.purplemath.com/modules/solvrtnl3.htm
Select a Course Below
Standardized Test Prep
Homeschool Math
© 2024 Purplemath, Inc. All right reserved. Web Design by ![]()



